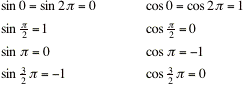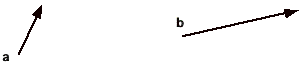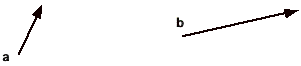Calculus Review
Part I
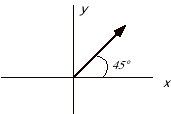
Review Basic Trig:
|
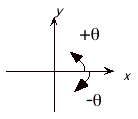
angle direction in the xy-plane defined: |
|
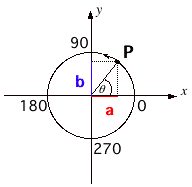
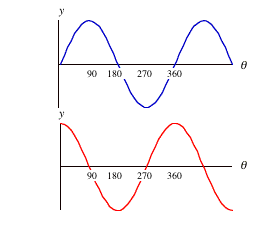
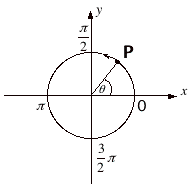
as point P rotates around the circle in the +q direction, the projection (or shaddow) of P's length along the x-direction (line a) and its projection along the y-direction (line b) trace out the following shapes:
|
|
|
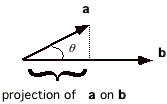
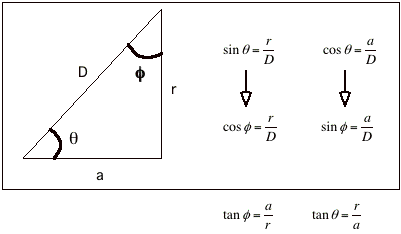
The projection of P on the x-axis as Pmoves
along the circle (labeled a in the previous diagram) is the ![]() and the
projection of Pon the y-axis as Pmoves
along the circle is the
and the
projection of Pon the y-axis as Pmoves
along the circle is the ![]() .
.
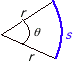
As you will see, it is often more
convenient to use radians rather than
degrees. There are 2p radians in a
circle. For example, consider the
arc s of a circle with
radius r. If q
is in radians, then the arclength s is equal to ![]() . So if
. So if ![]() , then s = q.
, then s = q.
|
|
if the length from the origin of the circle to point P is equal to 1, then:
|
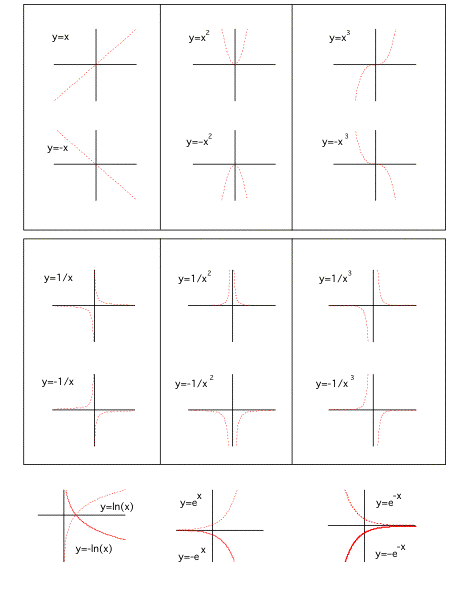
know the shapes of basic functions:
Practice
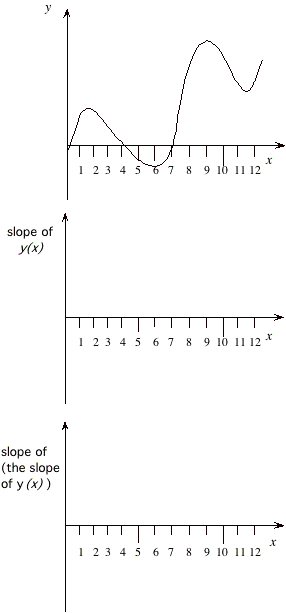
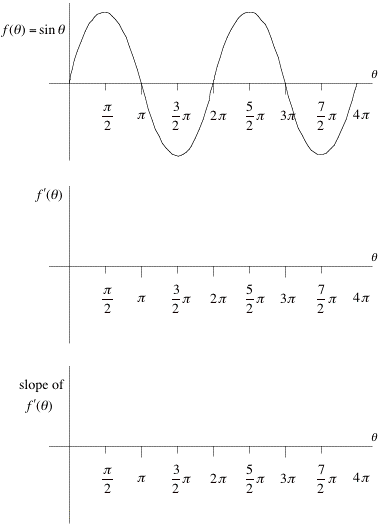
drawing the slope of various functions by eye:
|
in the middle panel, draw the slope of the curve on top, y(x). First sketch the slope at a few points of interest and then connect them. To do this, first find where the slope of y(x) is flat (=0) and mark the points on the plot below. Then by looking at the slope of y(x) on both sides of the flat points, sketch the curve by connecting the dots. now plot the slope of the curve above in the same manner |
|
In part, the calculus is the study of slopes:
|
|
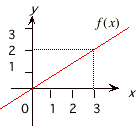
the function = in the
notation of the calculus, the slope of |
?
What is the slope of ![]() at x=2?
at x=2?
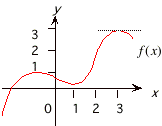
Newton was interested in the slopes of more complicated functions, for example, polynomials:
|
|
? What is the slope of ? What is the slope of |
Although for a straight line the slope is the same (constant) at all point on the line, this is not the case for polynomials.
How do you find
the slope of ![]() at any given x?
at any given x?
|
Use the formula: |
|
|
Find the slope
of ![]()
![]()
?
What is the slope of ![]()
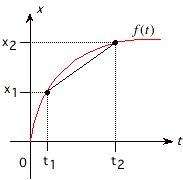
Newton invented the calculus because he was interested in velocity (Leibnitz also formulated the calculus independently). Velocity is displacement (x) per time (t).
Consider a ball moving along the x axis.
|
|
from
= average slope = average velocity |
Newton asked, what is the instantaneous velocity at some ![]() ?
?
but at one instant, ![]() and
and ![]() so the
velocity =
so the
velocity = ![]() !!!!!
!!!!!
So how do we get the slope at ONE POINT?
Newton and Leibniz invented the idea of a LIMIT , where the basic idea is that you sneak up on the point you're interested in but never quite get there.
HOW
A LIMIT GIVES THE FORMULA FOR THE SLOPE OF A FUNCTION
|
|
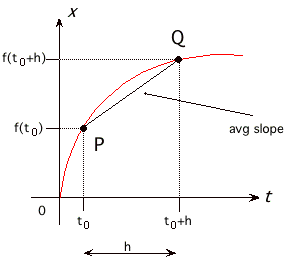
You want to know the velocity of the ball when it is at point P. Consider a
very, very small number, h. You can calculate the average
velocity (slope) of the ball between time |
|
||
|
So let's start with the average slope between P and Q:
and if you do the algebra, in the denominator, you'll get:
|
|||
|
|
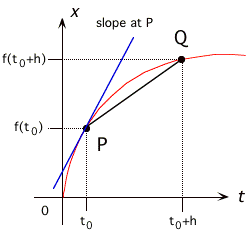
Now, hold P fixed, but make h become smaller and smaller. This has the effect of moving Q closer to P. |
|||
|
|
Notice how as h gets smaller, the average slope from P to Q approaches the slope exactly at P. |
|||
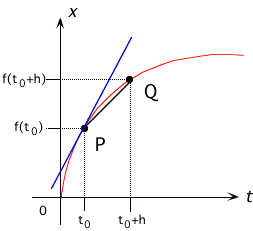
This is the idea of a LIMIT. As h gets closer and closer to 0, Q gets closer to P. We're using h to sneak up on P. Mathematically we can say that in the limit that h approaches 0, the average slope between P and Q approaches the instantaneous slope at P:
![]() and then we can
rewrite
and then we can
rewrite ![]()
where d is a sign for "differential" meaning a very, very small D. The mathematical definition of a DERIVATIVE is:
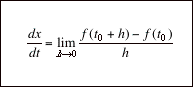
so the instantaneous velocity at P, ![]() , is not
, is not ![]() since although h gets very close to 0, h is never equal to 0!
Here are two different ways of indicating the slope of a function:
since although h gets very close to 0, h is never equal to 0!
Here are two different ways of indicating the slope of a function:
![]()
Now let's apply the definition to
a real function to see what happens.
Apply it to ![]() .
.
![]()
![]()
![]()
![]()
And if we now let h equal 0, we get: ![]()
Then the slope at any value of t for ![]() is
is ![]()
If you continue to use the definition of a derivative for other polynomials, you will soon see a pattern. You will find that for any value of n, and where a is some constant not equal to 0, if:
|
then |
or, if x is the independent variable such that ![]() ,
,
|
then |
?
What is the slope at ![]()
?
What is the slope at ![]()
?
![]() What is the slope
of
What is the slope
of ![]() ? At what x is
? At what x is ![]() flat?
flat?
As you can see, the slope of any polynomial is a function of one degree less than the original function. What about trig functions....
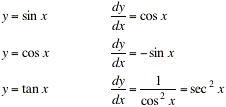
Know these by heart, but first, convince yourself that these are true (see next page)
?
![]() At
what x is the slope of
At
what x is the slope of ![]() equal to 0?
equal to 0?
|
Start by drawing the slope of Now draw the slope of the function in the middle panel. This is also a new function. What is it? |
|
Now let's look at velocity.
|
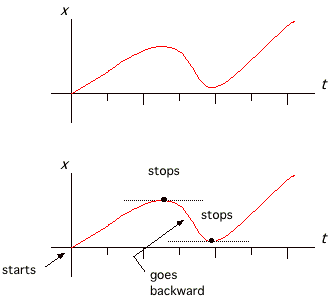
Imagine an object that moves in one direction (call it the x-direction). A plot of its motion in x with time looks like this: Notice that this curve looks
like a cubic polynomial, so you should expect that the displacement is a
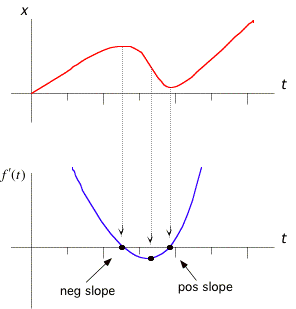
function of Consider what the slope of the displacement curve tells you about the motion. A positive slope means the object is increasing its distance from the starting point, a flat slope means the object has stopped, and a negative slope means the object has turned around and headed back toward the starting point. |
|
|
Then these points on the
displacement curve are points of interest. Plot the slope at
these points on the graph of This plot is a graph of the how the displacement changes in time. A change of displacement with time we defined as the velocity. The derivative of the displacement is the velocity! Notice that since the object
stops and then goes backward and stops and then goes forward, there must be a
place inbetween the two stops where the object turns around. Note also that the shape of the
velocity is parabolic; it goes as |
|
|
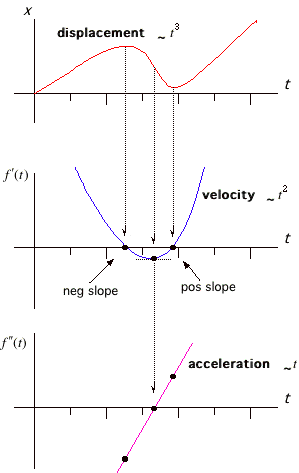
Now draw the slope of the slope of the velocity curve to see how velocity changes with time. A change in velocity with time is defined to be acceleration. |
|
So, if the only information you have is that the displacement of an object is cubic, then you automatically know that the acceleration of the object must be linearly increasing in time! This is the power of the calculus.
If ![]() is the
displacement with time, then the first derivative of
is the
displacement with time, then the first derivative of ![]() is
is ![]() and is the
velocity of the object, and the second derivative of
and is the
velocity of the object, and the second derivative of ![]() is
is ![]() and is the
acceleration.
and is the
acceleration.
Let's
look at the example of motion caused by gravity.
First, drop a ball from rest off the top of a tall building. Measure its velocity at several points on its way to the ground (this could be done with a camera and timer or a laser trip with a timer).
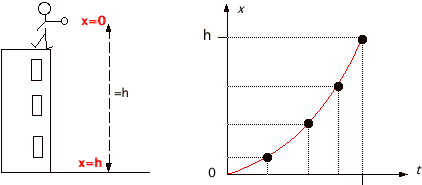
The measured points, x and t, will give a parabolic shape:
![]()
![]()
where k is a constant of proportionality. And then the velocity is linear:
![]()
![]()
and then the acceleration due to gravity is a constant:
![]()
![]()
Therefore, Newton found that all objects fall at the same (constant) rate!
Vectors
and Scalars
a scalar is a quantity which indicates an amount, for example, temperature, energy
a vector is a quantity that includes an amount and a direction.
A vector quantity is indicated by
having an arrow or half-arrow over the variable ![]() (handwriting)
(handwriting)
or in most text books, vectors
are printed in boldface,
![]() .
.
Vectors can be added or subtracted. Addition and subtraction of vectors, as for scalars, are commutative and associative.
Vectors can be manipulated geometrically or algebraically. Let's start with geometry.
Consider two vectors, a and b. Each has a magnitude (numerical amount) and a direction. The length of a vector is related to its magnitude.
|
|
Vector b has
a greater magnitude than a. |
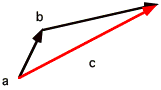
To add these vectors, place the tail of one at the head of the other, and draw a new vector to connect:
|
|
here,
|
Let's do it the other way:
|
|
here,
|
so vector addition is commutative.
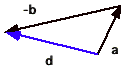
To subtract, take one vector and flip it around:
|
start with:
now flip b:
|
|
|
|
Now add a to -b as before to get
|
|
|
Or, if you want
then flip vector a
around before adding to b |
Notice that although the direction of the resulting vectors above are opposite each other, their magnitudes (lengths) are the same.
The way to indicate the magnitude
only of a vector, put it in between parallel lines: ![]() means the
magnitude (length) of vector c.
means the
magnitude (length) of vector c.
|
Plotting a vector requires knowing its magnitude and a angle it makes with respect to some axis. Usually you will be given an angle with respect to the x-axis, going counterclockwise, as is the convention. So, plot a vector 2 inches in magnitude, with q=45¡ from the x-axis. |
|
Another way to work with vectors is algebraically, which is much easier than having to draw and measure lines on a graph!
The idea is that any vector can be divided up into the part that projects on the x-axis and the part that projects on the y-axis. So a vector can be expressed as a sum of the x-part and the y-part.
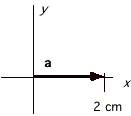
Consider a vector in the xy-plane a. It has magnitude of 2 cm lies completely along the x-axis (so the angle it makes with x is 0). This is the geometrical description.
|
|
The algebraic or
"component" description of this vector is: |
|
|
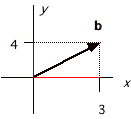
Another vector is: What is the length of b? From Phythagorus,
|
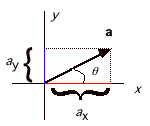
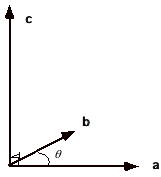
In general, any vector can be written as the sum of its projections along all axis of a given coordinate system, that is,
![]()
Notice that ![]() are lengths, NOT
vectors. The magnitude (total
length) of vector a is:
are lengths, NOT
vectors. The magnitude (total
length) of vector a is:
![]()
And notice that there are no direction vectors in the equation for the magnitude of a.
|
|
The component of a on the x-axis is
where
and |
To add vectors by components, you sum the like-components:
![]() and
and ![]()
Vector
multiplication
1) Scalar multiplication (also called the dot product)
When you multiply two vectors to get a dot product, you simply multiply like components and then sum the result. The result of scalar multiplitcation is a scalar, NOT a vector!
![]()
another way to get the same answer is to multiply the magnitude of each vector together and then multiply the result by the cosine of the angle between the vectors:
![]()
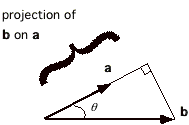
this second way of defining the dot product should help you visualize what the dot product really is: it is the magnitude of first vector multiplied by the projection of the second vector on the first:
|
|
= |
|
both of the above are equal to ![]() , that is, scalar multiplication of vectors is commutative.
, that is, scalar multiplication of vectors is commutative.
Also notice what happens if ![]() . In that case,
neither vector has a projection on the other. Therefore, if two vectors are at right angles to each other
(orthogonal), their dot product is zero.
This has great physical significance. An example is work.
. In that case,
neither vector has a projection on the other. Therefore, if two vectors are at right angles to each other
(orthogonal), their dot product is zero.
This has great physical significance. An example is work.
![]() Notice
that W is a scalar. So work is the component of the force
in the x-direction times how far
the object moves. If the angle
between the applied force and the direction you want the mass to move is 90¡, then you're pushing down when you want the
mass to go sideways, and so no work is done.
Notice
that W is a scalar. So work is the component of the force
in the x-direction times how far
the object moves. If the angle
between the applied force and the direction you want the mass to move is 90¡, then you're pushing down when you want the
mass to go sideways, and so no work is done.
2) Vector product (also
called the cross product)
Another method to multiply two vectors is called the vector product, because the result of the multiplication is another vector! This one isn't as easy to visualize, but it, too, has important physical application. If you want to try to picture it, try this: the vector product is the perpendicular projection of the first vector on the second, multiplied by the magnitude of the second vector, and pointing in a direction that is at right angles (orthogonal) to both vectors:
![]()
|
|
Here, Therefore, vector
multiplication is NOT commutative.
|
The way to carry out vector multiplication by components is a bit complicated, but once you get the hang of it, it isn't too bad. (The method will help you when you take linear algebra!)
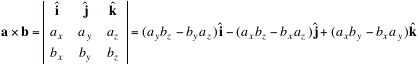
to get the x-component of vector c:
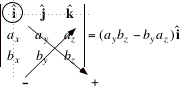
to get the y-component of vector c (notice the neg sign in front):
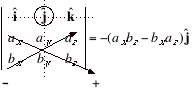
and finally to get the z-component of vector c:
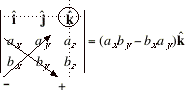
Notice that if ![]() were parallel to
each other, the vector product would be zero. The vector multiplication of an electric field vector with a
magnetic field vector, tells us the direction and strength of the propagation
of light.
were parallel to
each other, the vector product would be zero. The vector multiplication of an electric field vector with a
magnetic field vector, tells us the direction and strength of the propagation
of light.
2nd
Derivative
The derivative of a derivative is called the second derivative and can be written as:
![]()
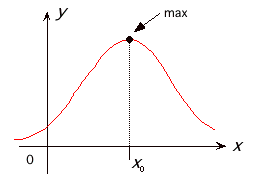
The second derivative can be used
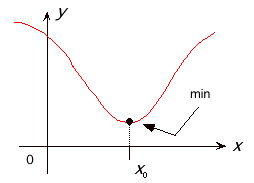
to find the minimum or maximum point on ![]() .
.
|
|
if has a local maximum at this point. |
|
|
if has a local minimum at this point. |
example:
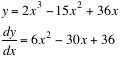
now find where the derivative is equal to zero.

and therefore ![]() is flat at two
points:
is flat at two
points: ![]()
now find if these points are min
or max by looking at the 2nd derivative evaluated at ![]() .
.
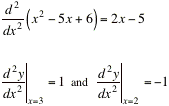
therefore ![]() has a min at
has a min at ![]() .
.
Techniques
of Differentiation
1) complicated polynomials:
in general,
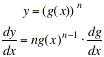
for example:
![]()
![]()
2) Products of polynomials
![]()
the rule is:
![]()
for example:
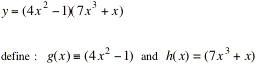
![]()
and then: ![]()
3) Trig functions raised to powers:
example:
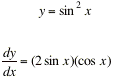
4) Chain rule:
![]()
then
let ![]()
we can rewrite y in terms of u:
![]()
and so
![]()
so then we have
![]()
ANSWERS
page 5:
|
? What is the slope of |
flat (zero) |
|
? What is the slope of |
steep and positive |
|
? What is the slope of |
|
page 9:
|
? What is the slope at |
|
|
? What is the slope at |
|
|
? |
|
|
? |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|